When it comes to arithmetic, commutative law or commutative property states that the order of terms does not matter while performing arithmetic operations.
Multiplication and addition are related, the commutative property only applies to these processes. This basically means that we can change the position or swap the numbers when adding or multiplying any two numbers. Integers are one of the most important of all numbers.
For example: 1+2 = 2+1 and 2 x 3 = 3 x 2.
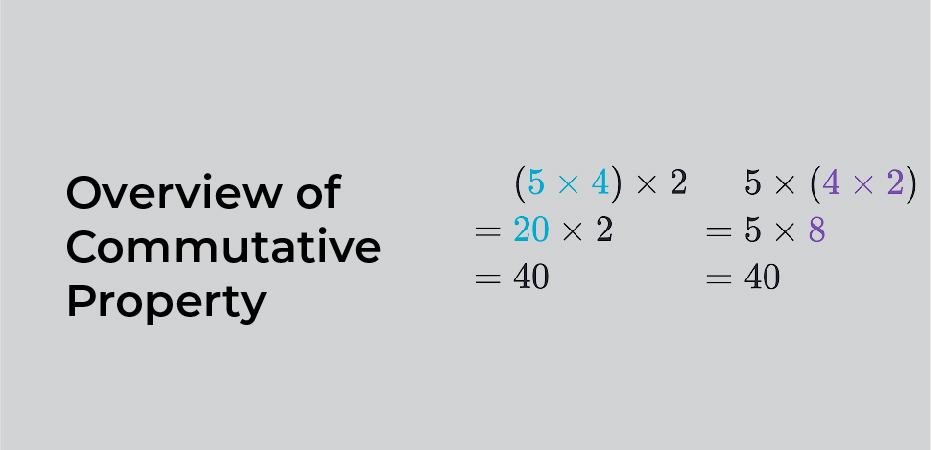
Overview of Commutative Property
As we already discussed in the introduction, according to commutative property or commutative law, if two numbers are added or multiplied together, then a change in their relative positions will not have any effect on the result.
Examples
- 2+3 = 3+2 = 5
- 2 x 3 = 3 x 2 = 6
- 5 + 10 = 10 + 5 = 15
- 5 x 10 = 10 x 5 = 50
In this sense, there can be two types of operations that obey the commutative property:
- Commutative property of addition
- Commutative property of multiplication
Background History
In spite of the fact that commutative property was first used formally at the end of the 18th century, it was known even in ancient times.
Commutative derives from the French word commuter, which means to switch or move around, and the suffix -active refers to the act or quality of tending. Accordingly, the literal meaning of the word is to switch from one place to another. As a result, swiping the positions of the integers will have no effect.
- Commutative Property of Addition
When we add two integers, the answer remains unchanged regardless of the position of the numbers, in accordance with the commutative property of addition.
Let A and B be the two integers, then;
- A + B = B + A
Examples of Commutative Property of Addition
- 1 + 2 = 2 + 1 = 3
- 3 + 8 = 8 + 3 = 11
- 12 + 5 = 5 + 12 = 17
- Commutative Property of Multiplication
Multiplication has the commutative property and as a result, when we multiply two integers, the answer we get after multiplication will remain the same, even if the positions of the integers are changed.
Let A and B be the two integers, then;
- A × B = B × A
Examples of Commutative Property of Multiplication
- 1 × 2 = 2 × 1 = 2
- 3 × 8 = 8 × 3 = 24
- 12 × 5 = 5 × 12 = 60
- Associative Property of Addition and Multiplication
In accordance with the law of associative association, no matter how the numbers are grouped, you can add or multiply them together, and the answer will be the same. It is important to note that the placement of parentheses does not matter in addition or multiplication when they are used to add or multiply.
Hence,
- A + (B + C) = (A + B) + C
- (B.C) = (A.B).C
Examples:
- 1 + (2+3) = (1+2) + 3 → 6
- 3 x (4 x 2) = (3 x 4) x 2 → 24
- Distributive Property of Multiplication
Essentially, the distributive property of Multiplication states that multiplying each addend by the value is the same as multiplying the sum by the value and adding the products together.
For instance, if a, b, c are certain real numbers, then the distributive property says that if they are:
a x (b + c) = (a x b) + (a x c)
Example:
- 2 x (5 x 8) = (2 x 5) + (2 x 8) → 80
Additionally, integers have other properties such as Identity property and Closure property.